MOTION PROBLEM INVOLVING LINEAR EQUATIONS IN ONE VARIABLE

Two jeepneys starting from the same place and traveling in opposite directions are 528 kilometers apart in 6 hours. One rate is 7 kilometers per hour faster than the other rate. Find the rate of each.
 
Solution:
 
Let x = rate of one jeepney
x + 7 = rate of the other jeepney
 
Based from the distance formula, D = rt, where D is the distance, r is the rate and t is the time, then
 
6x + 6(x + 7) = 528                    ] equation
6x + 6x + 42 = 528
12x = 528 - 42
12x = 486
12      12
 
x = 40.5 kms per hour             ] speed of one jeepney
x + 7 = 47.5 kms per hour      ] speed of the other jeepney
 
Hence, one jeepney was traveling at 40.5 km/hr while the other jeepney was traveling at 47.5 km/hr.
 
Check:
 
6(40.5) + 6(40.5 + 7) = 528
243 + 6(47.5) = 528
243 + 285 = 528
528 = 528

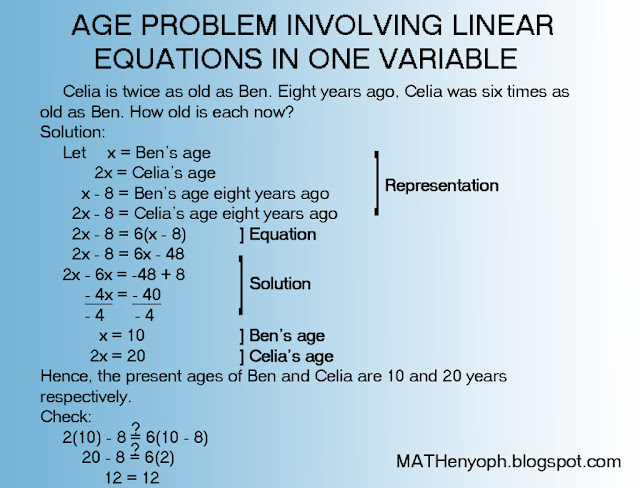
AGE PROBLEM INVOLVING LINEAR EQUATIONS IN ONE VARIABLE

Celia is twice as old as Ben. Eight years ago, Celia was six times as
old as Ben. How old is each now?
 
Solution:
 
Let x= Ben’s age
2x = Celia’s age
 x - 8 = Ben’s age eight years ago
2x - 8 = Celia’s age eight years ago
2x - 8 = 6(x - 8)                - equation
2x - 8 = 6x - 48
2x - 6x = -48 + 8
- 4x = - 40
- 4      - 4
 
x = 10                   - Ben's age
 
2x = 20                - Celia's age
 
Hence, the present ages of Ben and Celia are 10 and 20 years respectively.
 
Check:
 
2(10) - 8 = 6(10 - 8)
20 - 8 = 6(2)
12 = 12

COIN PROBLEM INVOLVING LINEAR EQUATIONS IN ONE VARIABLE

Mary has P10.50 in twenty-five centavo coins and ten centavo coins. She has 7 more ten centavo coins than twenty-five centavo coins. How many coins of each kind does she have?

Solution:

Let x = number of twenty-five centavo coins
x + 4 = number of ten centavo coins
.25x + .10(7 + x) = 10.50            - equation
100 [.25x + .10(7 + x)] = 10.50(100)
25x + 10(7 + x) = 1050
25x + 10x = 1050 - 70
35x = 980
35       35
x = 28                  - number of twenty-five centavo coins
x + 7 = 35            - number of ten centavo coins

Hence, there are 28 twenty-five centavo coins and 35 ten centavo coins amounting to P10.50.

Check:
.25(28) + .10(35) = 10.50
7 + 3.5 = 10.50
10.50 = 10.50

NUMBER PROBLEM INVOLVING LINEAR EQUATIONS IN ONE VARIABLE

One number is four greater than the other. The sum of the two numbers is 43. Find the numbers.

Solution:

Let x = smaller number
x + 4 = larger number
x + (x+4) = 43     - equation
2x + 3 = 43
2x = 43 - 3
2x = 40
2       2
x = 20                 - smaller number
x + 4 = 24           - larger number

Hence the number, 43, is the sum of the smaller number, 20 and the larger number 24.

Check:
20 + (20 + 4) = 43
43 = 43

MEASURES OF CAPACITY

10 milliliters (mL) = 1 centiliter (cL)
10 centiliters (cL) = 1 deciliter (dL)
10 deciliters (dL) = 1 liter (L)
10 liters (L) = 1 decaliter (DL)
10 decaliters (DL) = 1 hectoliter (HL)
10 hectoliters (HL) = 1 kiloliter (KL)
3 liters (L) = 1 ganta
25 gantas = 1 cavan
75 liters (L) = 1 cavan

MEASURES OF WEIGHT

10 milligrams (mg) = 1 centigram (cg)

10 centigrams (cg) = 1 decigram (dg)

10 decigrams (dg) = 1 gram (g)

10 grams (g)= 1 decagram (Dg)

10 decagrams (Dg) = 1 hectogram (Hg)

10 hectograms (Hg) = 1 kilogram (kg)

1,000 kilograms (kg) = 1 metric ton (MT)

MEASURES OF VOLUME

1,000 cubic millimeters (mm3) = 1 cubic centimeter (cm3)
1,000 cubic centimeters (cm3) = 1 cubic decimeter (dm3)
1,000 cubic decimeters (dm3) = 1 cubic meter (m3)
1,000,000,000 cubic centimeters (cm3) = 1 cubic meter (m3)

MEASURES OF AREA

100 square millimeters (mm2) = 1 square centimeter (cm2)
100 square centimeters (cm2) = 1 square decimeter (dm2)
100 square decimeters (dm2­) = 1 square meter (m2)
100 square meters (m2) = 1 square decameter (dkm2)
100 square decameters (dkm2) = 1 square hectometer (hm2)
100 square hectometers (hm2) = 1 square kilometer (km2)
10,000 square centimeters (cm2) = 1 square meter (m2)
 1,000,000,000 square meters (m2) = 1 square kilometer (km2)
10,000 square meters (m2) = 1 hectare (ha)

MEASURES OF LENGTH

10 millimeters (mm) = 1 centimeter (cm)
10 centimeters (cm) = 1 decimeter (dm)
10 decimeters (dm) = 1 meter (m)
10 meters (m) = 1 decameter (dkm)
10 decameters (dkm) = 1 hectometer (hm)
10 hectometers (hm) = 1 kilometer (km)
100 centimeters (cm) = 1 meter (m)
 1,000 meters (m) = 1 kilometer (km)

PERIMETER

Perimeter is the measure around a closed figure.
To find the perimeter of a polygon, add the measures of all sides.
Perimeter is measured in linear units such as centimeter, decimeter, meter, kilometer, etc.

Formulas to find the perimeter of:

1. Square
P = S1 + S2 + S3 + S4 or P = 4s

2. Rectangle
a. P = l + w + l + w
b. P = 2l + 2w
c. P = 2(l + w)

3. Triangle
 P = S1 + S2 + S3

4. Rhombus
P = S1 + S2 + S3 = S4 or P = 4s

*P = Perimeter*
*S = Side*
*l = length*
*w = width*

DIVISION OF DENOMINATE NUMBERS

1. Arrange the units, start with the highest unit at the left end.
2. Divide starting from the highest unit.
3. Add the remaining if there is any to the next lower unit.
(Be sure to change the remainder to the lower unit before adding)

Ex. James is going to cut his 20 feet 6 inches in rope into 6 equal pieces. How long will each piece be?

Solution:

Step 1. Arrange the units and write the divisor.
   ________
6 )20 ft 6 in

Step 2. Divide starting from the highest unit.

   _3ft_____
6 )20 ft 6 in
    18__
     2 ft

Step 3. Add the remainder to the lower unit. (Be sure to change the remainder to the lower unit before adding)

2 ft = 24 inches. So, 24 inches + 6 inches = 30 inches

Step 4. Divide the lower unit.

    _3ft  5 in_
6 )20 ft 6 in
    18__
     2 ft
            30 in ( step 3)
     -     30 in
             0

ANSWER: 3 feet 5 inches

MULTIPLICATION OF DENOMINATE NUMBERS

1. Arrange the units, start with the higher unit at the left end.
2. Write the multiplier in column with the smallest unit.
3. Multiply the numbers.
4. Reduce the answer to the higher denominations if necessary.
 
Ex. Chris studies his lessons everyday on the average of 2 hours 40 minutes. How long is it in 5 days?
 
Solution no. 1:
 
Step 1. Arrange the units and write the multiplier.
 
2 hrs 40 min
   x                 5
 
Step 2. Multiply starting from right.
 
40 min x 5 = 200 min
200 min = 3 hrs 20 min. So, write down 20 min and carry 3hrs.
 
3hrs
2hrs 30 min
    x               5
 
ANSWER: 13 hrs 20 min
 
Solution no. 2:
 
Step 1. Multiply.
 
2 hrs 40 min
    x                 5
10 hrs 200 min
 
Step 2. Reduce the answer to the higher term. 

200 min = 3 hrs 20 min So,
10 hrs 200 min = 13 hrs 20 min
 
ANSWER: 12 hours 30 minutes